Tippetop links

The tippetop

This page is set up by Dr. Maria-Cristina Ciocci (Howest), Dr. Benny Malengier (UGent, Dr. Bavo Langerock and Dhr. Bart Grimonprez (Howest). We have been working on an article TOWARDS A PROTOTYPE OF A SPHERICAL TIPPE TOP. On this page we present animations of the Tippe Top as a background for the article.

Tippe Tops can be classified in 3 main groups, 6 subgroups, called Ia, Ib, IIa, IIb, IIc and III. Some have the tipping behavior found in the common toy, some don't.

Info about the model

Different models exist for the tippetop. We analyse the sliding model, in which the tippetop rolls and slides over a flat surface. The tippetop is considered to be an eccentric (or loaded) sphere. Therefore, we show in the animations a glass sphere with an embedded top.

The type IIb tippe top

Properties

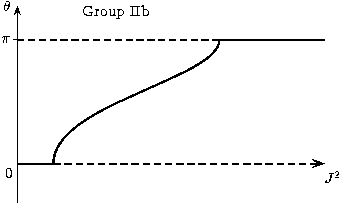The type IIb tippe top is characterized by the non-inverted state being stable up to an initial spin n1, where a branch of stable intermediate states comes into existence. This branch connects to the tipped state where this state becomes stable.

The data used for the tippe top are: mass 6 gram, radius 1.5 cm, eccentricity 00%, A 0.00000082 kg/(m*m) and C 0.0000007 kg/(m*m). The value of the friction coefficient mu is 0.3.

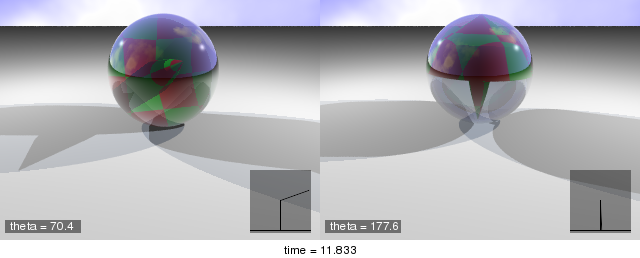
Animation of a complete flip

We show 90 seconds, which is presented as 7.5 minutes of film.

The animation is the mpeg2-format and has a file size of 16.4 MB. It can be started by clicking at the following preview picture:

Animation of movement to stable intermediate state

When the initial data is chosen to have a Jellet that coincides with a stable intermediate state, movement from theta=0 and theta=Pi towards the intermediate state is observed.

The animation is the mpeg2-format and has a file size of 32.7 MB. It corresponds to 45 seconds of the tippetop movement, with 5x slow motion. It can be started by clicking at the following preview picture.

The data of the tippetop did not change except for mu which is now 0.1. Furthermore, the initial conditions around theta 0 and Pi are chosen to correspond to a Jellet of approximately 0.84 10^-6. Movement towards the intermediate state at theta 134.5 degrees is observed.

The type IIa tippe top

Properties

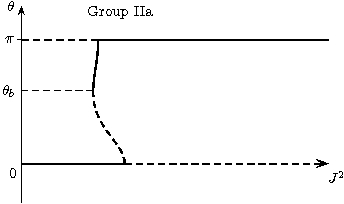The type IIa tippe top is characterized by the non-inverted state being stable up to an initial spin n1, after which total flip occurs. However, from n1, a branch of unstable intermediate states comes into existence. This branch becomes stable, and finally connects to the tipped state where this state becomes stable.

Therefore, it is possible to start in the flipped state, and have movement towards the stable intermediate state, or if this is not present for the chosen initial conditions, towards the stable non-inverted state

The data used for the tippe top are: mass 6 gram, radius 1.5 cm, eccentricity 50%, A 0.000000385 kg/(m*m) and C 0.0000007 kg/(m*m). The value of the friction coefficient mu is 0.08.

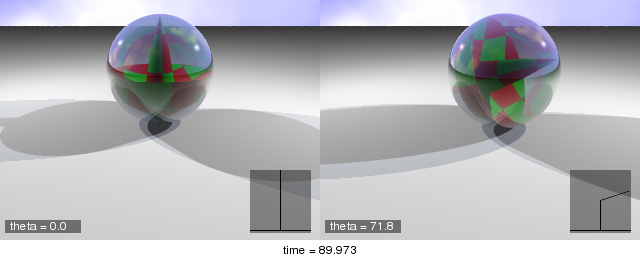
Animation of movement starting in unstable inverted state

In the left of the animation we see the behaviour in which the tippetop flips towards the non-inverted state. However, oscillation of theta occurs. To the right, we see the movement for a slightly different initial state, with movement towards an intermediate state. Also here oscillation of theta occurs.

The oscillation of theta is visible in the corresponding figure in the article, where the path is shown in n, theta, alpha coordinates.

Info about the animations

The files and scripts needed to make the animations will be made available on this webpage on publication of the manuscript. The procedure is as follows: a maple sheet is run that solves with a suitable ODE method the tippetop model. We chose to use 5x slow motion, so 1 second takes 5 seconds in the animation, with 30 frames for every second. This is done so as to see clearly the rotation of the tippetop.

The output of Maple is used in a script to create for every frame an image. This is done with the povray program. Another script is used to add data to the picture using Imagemagick. All the frames are glued together in a film with ffmpeg.

Coordinates

Maria-Cristina Ciocci
Antoon Catriestraat 6,
B-9031 Drongen, Belgium
email: <mcristina.ciocci@gmail.com>
phone: +32 (0)9 220 27 52