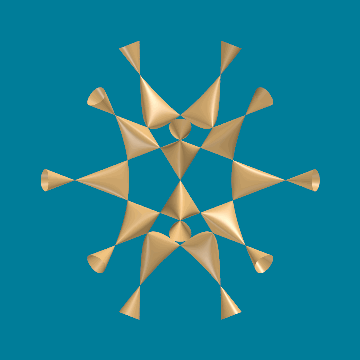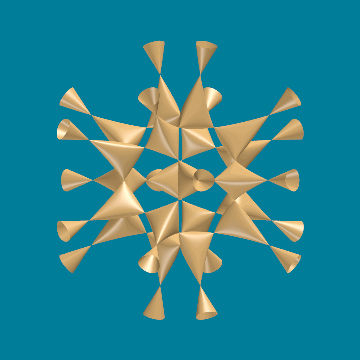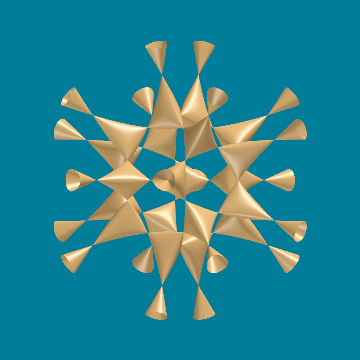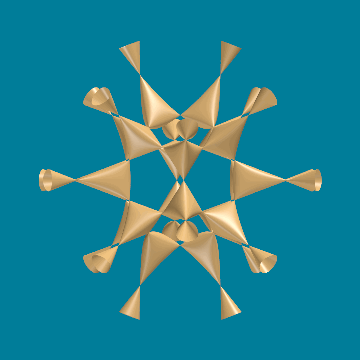
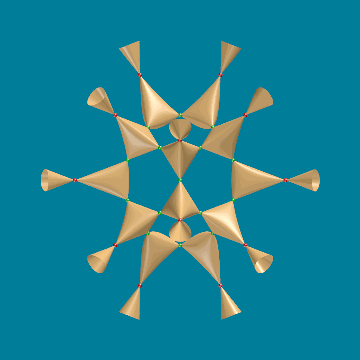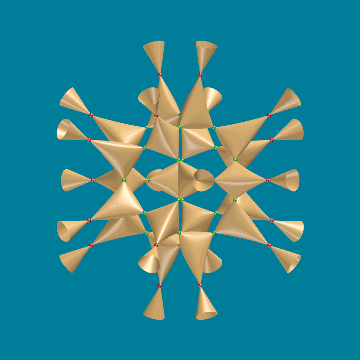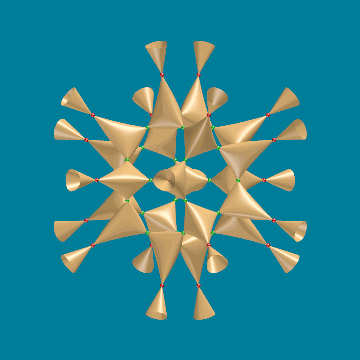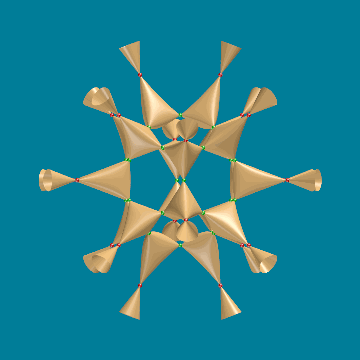
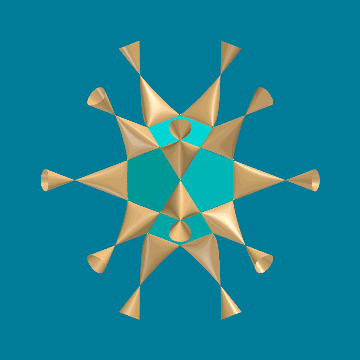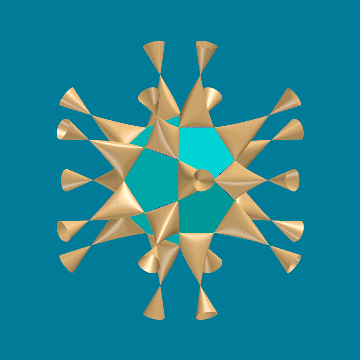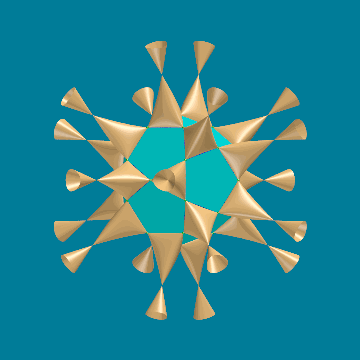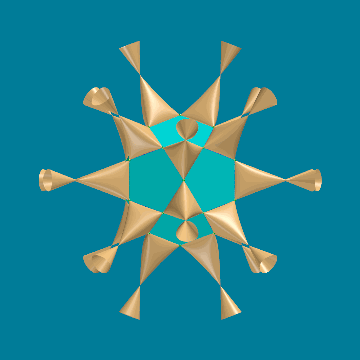
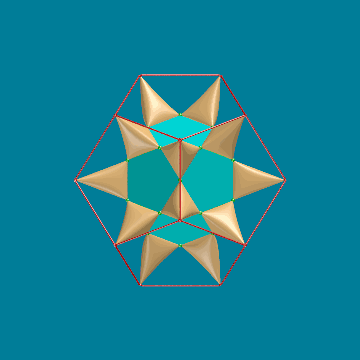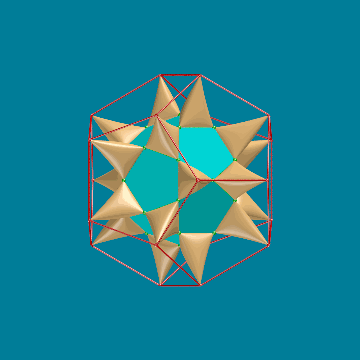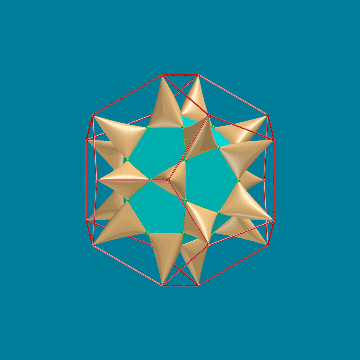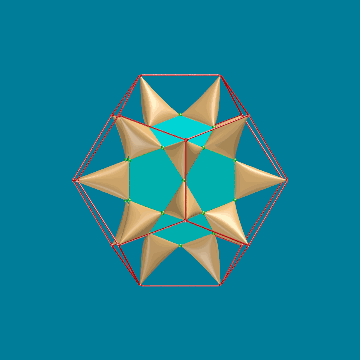
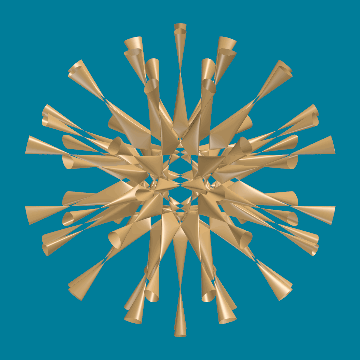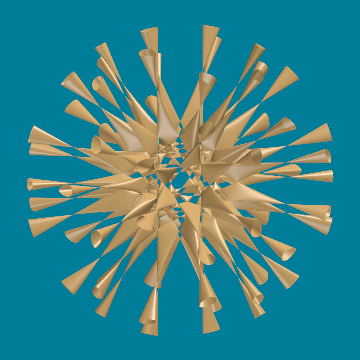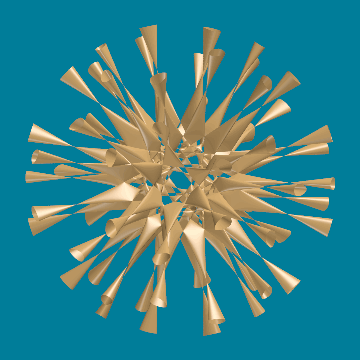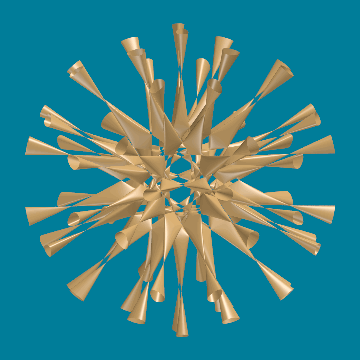
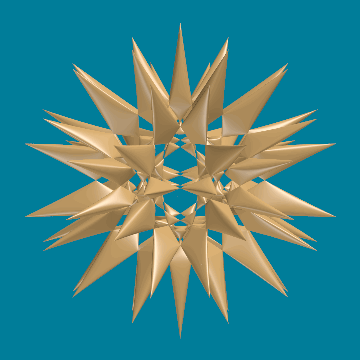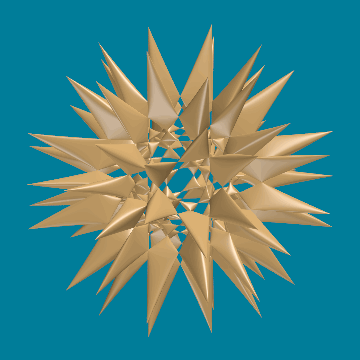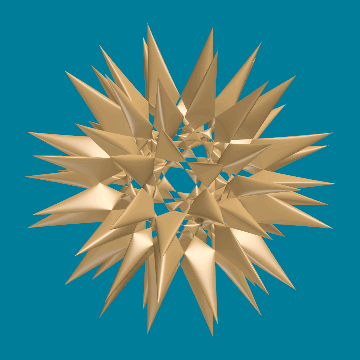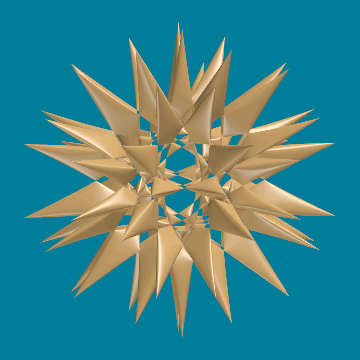
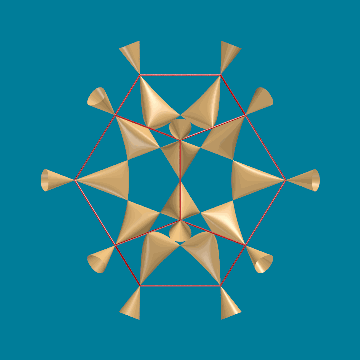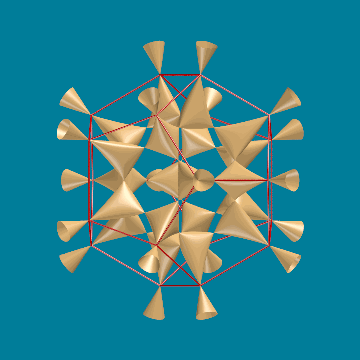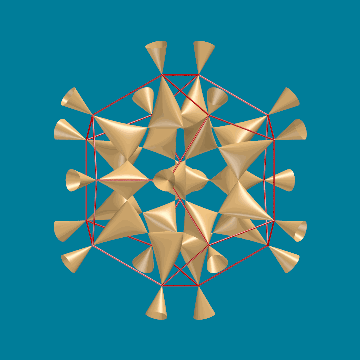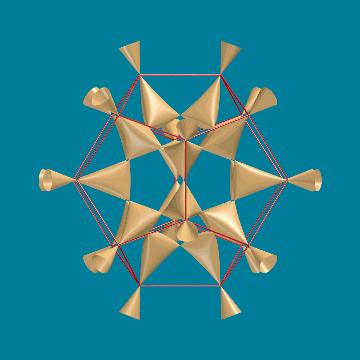Barth's Sextic Surface
Barth's sextic surface
is the surface determined by the polynomial equation
4(Φ2x2-y2)(Φ2y2-z2)(Φ2z2-x2) - (1+2Φ)(x2+y2+z2-1)2=0
Φ=(sqrt(5)+1)/2 is the golden number, appearing in the
golden section
The following animation shows the part of the surface inside a sphere centered at the origin.
Wolf Barth (1942-2016) was a German mathematician. In April 2017 Thomas Bauer, Klaus Hulek, Slawomir Rams, Alessandra Sarti and Tomasz Szemberg published an article consacrated to his life and work:
Wolf Barth 1942-2016.
As is written in this article: "Wolf Barth was interested in the visualization of algebraic surfaces. With the availability of ever more powerfull computers, it was his idea that, given the polynomial equation f(x,y,z)=0 of an algebraic surface,
it should be possible to generate convincing images of the real surface. He was fascinated by the beauty of the surfaces that one can produce using symmetries".
In 1996 he discovered what is now called Barth's sextic surface. It is a surface of degree 6 with 65 singularities, the maximum number of singularities possible for surfaces of degree 6.
50 singularities are real and visible as "nodes", the other 15 are "points at infinity".
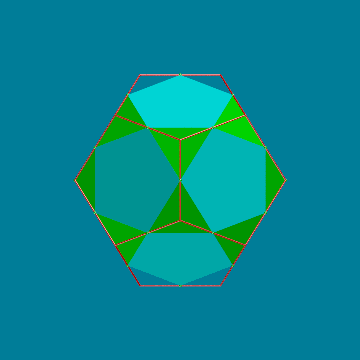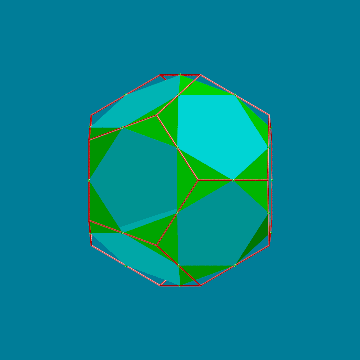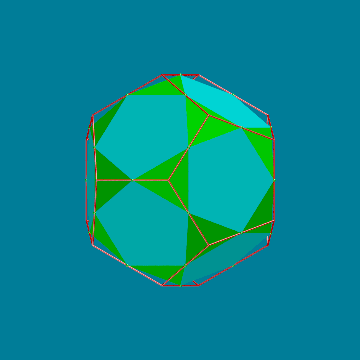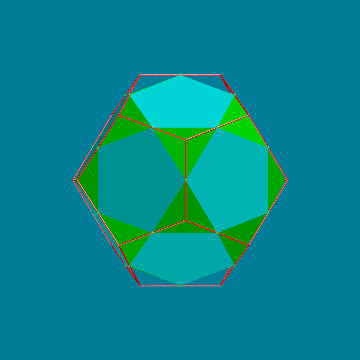
20 nodes (the red ones) are the vertices of a regular dodecahedron:
The other real 30 nodes (the green ones) are the vertices of an icosidodecahedron. An icosidodecahedron is one of the 13 Archimedean solids.
It can be constructed starting from a regular dodecahedron and cutting off the 20
pyramids determined by the vertices of the dodecahedron and the midpoints of its edges. An icosidodecahedron has 30 vertices, 32 faces (12 pentagons and 20 triangles) and 60 edges.
An icosidodecahedron can be constructed in the same way starting from a regular icosahedron.
Limiting the surface to the part within the sphere passing through the red nodes, we obtain the following animation:
Barth's sextic surface clipped by an expanding and shrinking sphere:
Barth's Decic Surface
Barth's decic surface
is the surface determined by the polynomial equation
8(x2-Φ4y2)(y2-Φ4z2)(z2-Φ4x2)
(x4+y4+z4-2x2y2-2y2z2-2z2x2)
+(3+5Φ)(x2+y2+z2-1)2
(x2+y2+z2-2+Φ)2=0
Φ=(sqrt(5)+1)/2 is the golden number, appearing in the
golden section.
Barth's decic surface has 345 singularities, 300 among them are real nodes.
The following animation shows the part of the surface inside a sphere centered at the origin.
Barth's decic surface clipped by an expanding and shrinking sphere:
Barth's decic surface clipped by a sphere passing through a number of equivalent nodes:
As mentioned in "Barth's decic surface", the nodes of the surface are associated with some more
sophisticated polyhedra.
Barth's original paper on his sextic and decic surface:
Barth, W: Two projective surfaces with many nodes, admitting the symmetries of the icosahedron
Journal of Algebraic Geometry, 5, 173-186 (1996)
Update - December 2022
Herman Serras
Send Email
return to the homepage